
SL Paper 2
This question is in two parts. Part 1 is about energy resources. Part 2 is about electric fields.
Part 1 Energy resources
A photovoltaic panel is made up of a collection (array) of photovoltaic cells. The panel has a total area of \({\text{1.3 }}{{\text{m}}^{\text{2}}}\) and is mounted on the roof of a house. The maximum intensity of solar radiation at the location of the panel is \({\text{750 W}}\,{{\text{m}}^{ - 2}}\). The panel produces a power output of 210 W when the solar radiation is at its maximum intensity.
The owner of the house chooses between photovoltaic panels and solar heating panels to provide 4.2 kW of power to heat water. The solar heating panels have an efficiency of 70%. The maximum intensity of solar radiation at the location remains at \({\text{750 W}}\,{{\text{m}}^{ - 2}}\).
Part 2 Electric fields
An isolated metal sphere is placed in a vacuum. The sphere has a negative charge of magnitude 12 nC.
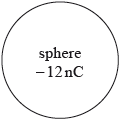
Outside the sphere, the electric field strength is equivalent to that of a point negative charge of magnitude 12 nC placed at the centre of the sphere. The radius \(r\) of the sphere is 25 mm.
An electron is initially at rest on the surface of the sphere.
The Sun is a renewable energy source whereas a fossil fuel is a non-renewable energy source. Outline the difference between renewable and non-renewable energy sources.
With reference to the energy transformations and the operation of the devices, distinguish between a photovoltaic cell and a solar heating panel.
Determine the efficiency of the photovoltaic panel.
State two reasons why the intensity of solar radiation at the location of the panel is not constant.
1.
2.
Calculate the minimum area of solar heating panel required to provide this power.
Comment on whether it is better to use a solar heating panel rather than an array of photovoltaic panels for the house. Do not consider the installation cost of the panels in your answer.
Using the diagram, draw the electric field pattern due to the charged sphere.
Show that the magnitude of the electric field strength at the surface of the sphere is about \(2 \times {10^5}{\text{ N}}\,{{\text{C}}^{ - 1}}\).
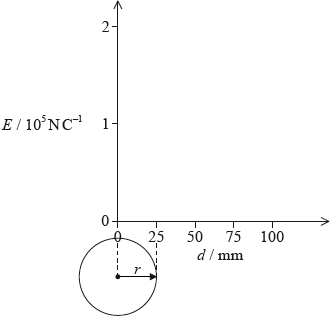
On the axes, draw a graph to show the variation of the electric field strength \(E\) with distance \(d\) from the centre of the sphere.
Calculate the initial acceleration of the electron.
Discuss the subsequent motion of the electron.
Define electric field strength.
The diagram shows a pair of horizontal metal plates. Electrons can be deflected vertically using an electric field between the plates.
(i) Label, on the diagram, the polarity of the metal plates which would cause an electron
positioned between the plates to accelerate upwards.
(ii) Draw the shape and direction of the electric field between the plates on the diagram.
(iii) Calculate the force on an electron between the plates when the electric field strength has a value of 2.5 × 103 NC–1.
The diagram shows two isolated electrons, X and Y, initially at rest in a vacuum. The initial separation of the electrons is 5.0 mm. The electrons subsequently move apart in the directions shown.
(i) Show that the initial electric force acting on each electron due to the other electron is approximately 9 × 10–24N.
(ii) Calculate the initial acceleration of one electron due to the force in (c)(i).
(iii) Discuss the motion of one electron after it begins to move.
(iv) The diagram shows Y as seen from X, at one instant. Y is moving into the plane of the paper. For this instant, draw on the diagram the shape and direction of the magnetic field produced by Y.
This question is in two parts. Part 1 is about a lightning discharge. Part 2 is about fuel for heating.
Part 1 Lightning discharge
The magnitude of the electric field strength \(E\) between two infinite charged parallel plates is given by the expression
\[E = \frac{\sigma }{{{\varepsilon _0}}}\]
where \(\sigma \) is the charge per unit area on one of the plates.
A thundercloud carries a charge of magnitude 35 C spread over its base. The area of the base is \(1.2 \times {10^7}{\text{ }}{{\text{m}}^{\text{2}}}\).
Part 2 Fuel for heating
A room heater burns liquid fuel and the following data are available.
\[\begin{array}{*{20}{l}} {{\text{Density of liquid fuel}}}&{ = 8.0 \times {{10}^2}{\text{ kg}}\,{{\text{m}}^{ - 3}}} \\ {{\text{Energy produced by 1 }}{{\text{m}}^{\text{3}}}{\text{ of liquid fuel}}}&{ = 2.7 \times {{10}^{10}}{\text{ J}}} \\ {{\text{Rate at which fuel is consumed}}}&{ = 0.13{\text{ g}}\,{{\text{s}}^{ - 1}}} \\ {{\text{Latent heat of vaporization of the fuel}}}&{ = 290{\text{ kJ}}\,{\text{k}}{{\text{g}}^{ - 1}}} \end{array}\]
Define electric field strength.
A thundercloud can be modelled as a negatively charged plate that is parallel to the ground.
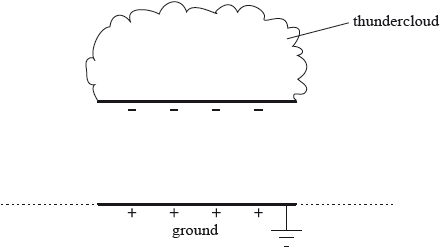
The magnitude of the charge on the plate increases due to processes in the atmosphere. Eventually a current discharges from the thundercloud to the ground.
On the diagram, draw the electric field pattern between the thundercloud base and the ground.
(i) Determine the magnitude of the electric field between the base of the thundercloud and the ground.
(ii) State two assumptions made in (c)(i).
1.
2.
(iii) When the thundercloud discharges, the average discharge current is 1.8 kA. Estimate the discharge time.
(iv) The potential difference between the thundercloud and the ground before discharge is \(2.5 \times {10^8}{\text{ V}}\). Determine the energy released in the discharge.
Define the energy density of a fuel.
(i) Use the data to calculate the power output of the room heater, ignoring the power required to convert the liquid fuel into a gas.
(ii) Show why, in your calculation in (b)(i), the power required to convert the liquid fuel into a gas at its boiling point can be ignored.
State, in terms of molecular structure and their motion, two differences between a liquid and a gas.
1.
2.
This question is in two parts. Part 1 is about electric charge and electric circuits. Part 2 is about momentum.
Part 1 Electric charge and electric circuits
State Coulomb’s law.
In a simple model of the hydrogen atom, the electron can be regarded as being in a circular orbit about the proton. The radius of the orbit is 2.0×10–10 m.
(i) Determine the magnitude of the electric force between the proton and the electron.
(ii) Calculate the magnitude of the electric field strength E and state the direction of the electric field due to the proton at a distance of 2.0×10–10 m from the proton.
(iii) The magnitude of the gravitational field due to the proton at a distance of 2.0×10–10 m from the proton is H.
Show that the ratio \(\frac{H}{E}\) is of the order 10–28C kg–1.
(iv) The orbital electron is transferred from its orbit to a point where the potential is zero. The gain in potential energy of the electron is 5.4×10–19J. Calculate the value of the potential difference through which the electron is moved.
An electric cell is a device that is used to transfer energy to electrons in a circuit. A particular circuit consists of a cell of emf ε and internal resistance r connected in series with a resistor of resistance 5.0 Ω.
(i) Define emf of a cell.
(ii) The energy supplied by the cell to one electron in transferring it around the circuit is 5.1×10–19J. Show that the emf of the cell is 3.2V.
(iii) Each electron in the circuit transfers an energy of 4.0×10–19 J to the 5.0 Ω resistor. Determine the value of the internal resistance r.
Part 2 Satellite
State, in words, Newton’s universal law of gravitation.
The diagram shows a satellite orbiting the Earth. The satellite is part of the network of global-positioning satellites (GPS) that transmit radio signals used to locate the position of receivers that are located on the Earth.
When the satellite is directly overhead, the microwave signal reaches the receiver 67ms after it leaves the satellite.
(i) State the order of magnitude of the wavelength of microwaves.
(ii) Calculate the height of the satellite above the surface of the Earth
(i) Explain why the satellite is accelerating towards the centre of the Earth even though its orbital speed is constant.
(ii) Calculate the gravitational field strength due to the Earth at the position of the satellite.
Mass of Earth = 6.0×1024kg
Radius of Earth = 6.4×106m
(iii) Determine the orbital speed of the satellite.
(iv) Determine, in hours, the orbital period of the satellite.
This question is in two parts. Part 1 is about momentum. Part 2 is about electric point charges.
Part 1 Momentum
Part 2 Electric point charges
State the law of conservation of linear momentum.
A toy car crashes into a wall and rebounds at right angles to the wall, as shown in the plan view.
The graph shows the variation with time of the force acting on the car due to the wall during the collision.
The kinetic energy of the car is unchanged after the collision. The mass of the car is 0.80 kg.
(i) Determine the initial momentum of the car.
(ii) Estimate the average acceleration of the car before it rebounds.
(iii) On the axes, draw a graph to show how the momentum of the car varies during the impact. You are not required to give values on the y-axis.
Two identical toy cars, A and B are dropped from the same height onto a solid floor without rebounding. Car A is unprotected whilst car B is in a box with protective packaging around the toy. Explain why car B is less likely to be damaged when dropped.
Define electric field strength at a point in an electric field.
Six point charges of equal magnitude Q are held at the corners of a hexagon with the signs of the charges as shown. Each side of the hexagon has a length a.
P is at the centre of the hexagon.
(i) Show, using Coulomb’s law, that the magnitude of the electric field strength at point P due to one of the point charges is
\[\frac{{kQ}}{{{a^2}}}\]
(ii) On the diagram, draw arrows to represent the direction of the field at P due to point charge A (label this direction A) and point charge B (label this direction B).
(iii) The magnitude of Q is 3.2 μC and length a is 0.15 m. Determine the magnitude and the direction of the electric field strength at point P due to all six charges.