
SL Paper 2
A farmer owns a field in the shape of a triangle such that and .
The local town is planning to build a highway that will intersect the borders of the field at points and , where and , as shown in the diagram below.
The town wishes to build a carpark here. They ask the farmer to exchange the part of the field represented by triangle . In return the farmer will get a triangle of equal area , where lies on the same line as and , as shown in the diagram above.
Find the size of .
Find .
Find the area of triangle .
Estimate . You may assume the highway has a width of zero.
Using geometry software, Pedro draws a quadrilateral . and . Angle and angle . This information is shown in the diagram.
, where point is the midpoint of .
Calculate the length of .
Show that angle , correct to three significant figures.
Calculate the area of triangle .
Pedro draws a circle, with centre at point , passing through point . Part of the circle is shown in the diagram.
Show that point lies outside this circle. Justify your reasoning.
The living accommodation on a university campus is in the shape of a rectangle with sides of length and .
There are three offices for the management of the accommodation set at the points , and . These offices are responsible for all the students in the areas closest to the office. These areas are shown on the Voronoi diagram below. On this coordinate system the positions of , and are , and respectively.
The equation of the perpendicular bisector of is .
The manager of office believes that he has more than one third of the area of the campus to manage.
Find the area of campus managed by office .
Hence or otherwise find the areas managed by offices and .
State a further assumption that must be made in order to use area covered as a measure of whether or not the manager of office is responsible for more students than the managers of offices and .
A new office is to be built within the triangle formed by , and , at a point as far as possible from the other three offices.
Find the distance of this office from each of the other offices.
The following diagram shows a circle with centre and radius . Points and lie on the circumference of the circle and , where .
The tangents to the circle at and intersect at point .
Show that .
Find the value of when the area of the shaded region is equal to the area of sector .
The diagram below shows a circular clockface with centre . The clock’s minute hand has a length of . The clock’s hour hand has a length of .
At pm the endpoint of the minute hand is at point and the endpoint of the hour hand is at point .
Between pm and pm, the endpoint of the minute hand rotates through an angle, , from point to point . This is illustrated in the diagram.
A second clock is illustrated in the diagram below. The clock face has radius with minute and hour hands both of length . The time shown is am. The bottom of the clock face is located above a horizontal bookshelf.
The height, centimetres, of the endpoint of the minute hand above the bookshelf is modelled by the function
where is the angle rotated by the minute hand from am.
The height, centimetres, of the endpoint of the hour hand above the bookshelf is modelled by the function
where is the angle in degrees rotated by the minute hand from am.
Find the size of angle in degrees.
Find the distance between points and .
Find the size of angle in degrees.
Calculate the length of arc .
Calculate the area of the shaded sector, .
Write down the height of the endpoint of the minute hand above the bookshelf at am.
Find the value of when .
Write down the amplitude of .
The endpoints of the minute hand and hour hand meet when .
Find the smallest possible value of .
A hollow chocolate box is manufactured in the form of a right prism with a regular hexagonal base. The height of the prism is , and the top and base of the prism have sides of length .
Given that , show that the area of the base of the box is equal to .
Given that the total external surface area of the box is , show that the volume of the box may be expressed as .
Sketch the graph of , for .
Find an expression for .
Find the value of which maximizes the volume of the box.
Hence, or otherwise, find the maximum possible volume of the box.
The box will contain spherical chocolates. The production manager assumes that they can calculate the exact number of chocolates in each box by dividing the volume of the box by the volume of a single chocolate and then rounding down to the nearest integer.
Explain why the production manager is incorrect.
A large water reservoir is built in the form of part of an upside-down right pyramid with a horizontal square base of length metres. The point is the centre of the square base and point is the vertex of the pyramid.
The bottom of the reservoir is a square of length metres that is parallel to the base of the pyramid, such that the depth of the reservoir is metres as shown in the diagram.
The second diagram shows a vertical cross section, , of the reservoir.
Every day of water from the reservoir is used for irrigation.
Joshua states that, if no other water enters or leaves the reservoir, then when it is full there is enough irrigation water for at least one year.
Find the angle of depression from to .
Find .
Hence or otherwise, show that the volume of the reservoir is .
By finding an appropriate value, determine whether Joshua is correct.
To avoid water leaking into the ground, the five interior sides of the reservoir have been painted with a watertight material.
Find the area that was painted.
The Tower of Pisa is well known worldwide for how it leans.
Giovanni visits the Tower and wants to investigate how much it is leaning. He draws a diagram showing a non-right triangle, ABC.
On Giovanni’s diagram the length of AB is 56 m, the length of BC is 37 m, and angle ACB is 60°. AX is the perpendicular height from A to BC.
Giovanni’s tourist guidebook says that the actual horizontal displacement of the Tower, BX, is 3.9 metres.
Use Giovanni’s diagram to show that angle ABC, the angle at which the Tower is leaning relative to the
horizontal, is 85° to the nearest degree.
Use Giovanni's diagram to calculate the length of AX.
Use Giovanni's diagram to find the length of BX, the horizontal displacement of the Tower.
Find the percentage error on Giovanni’s diagram.
Giovanni adds a point D to his diagram, such that BD = 45 m, and another triangle is formed.
Find the angle of elevation of A from D.
A sector of a circle, centre and radius , is shown in the following diagram.
A square field with side has a goat tied to a post in the centre by a rope such that the goat can reach all parts of the field up to from the post.
[Source: mynamepong, n.d. Goat [image online] Available at: https://thenounproject.com/term/goat/1761571/
This file is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported (CC BY-SA 3.0)
https://creativecommons.org/licenses/by-sa/3.0/deed.en [Accessed 22 April 2010] Source adapted.]
Let be the volume of grass eaten by the goat, in cubic metres, and be the length of time, in hours, that the goat has been in the field.
The goat eats grass at the rate of .
Find the angle .
Find the area of the shaded segment.
Find the area of a circle with radius .
Find the area of the field that can be reached by the goat.
Find the value of at which the goat is eating grass at the greatest rate.
A king rules a small mountain kingdom which is in the form of a square of length 4 kilometres. The square is described by the co-ordinate system .
The king has four adult children, each of which has a luxury palace located at the points . Each child owns all the land that is nearer their palace than any other palace.
The king has a fifth (youngest) child who is now just growing up. He installs her in a new palace situated at point (2, 2). The rule about ownership of land is then reapplied.
Sketch a Voronoi diagram to represent this information.
Sketch a new Voronoi diagram to represent this new situation.
State what the shape of the land, owned by the youngest child, is.
Find the area of the youngest child’s land.
Find how much land an older child has lost.
State, with a reason, if all five children now own an equal amount of land.
The Voronoi diagram below shows four supermarkets represented by points with coordinates , , and . The vertices , , are also shown. All distances are measured in kilometres.
The equation of is and the equation of is .
The coordinates of are and the coordinates of are .
A town planner believes that the larger the area of the Voronoi cell , the more people will shop at supermarket .
Find the midpoint of .
Find the equation of .
Find the coordinates of .
Determine the exact length of .
Given that the exact length of is , find the size of in degrees.
Hence find the area of triangle .
State one criticism of this interpretation.
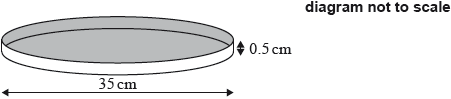
A pan, in which to cook a pizza, is in the shape of a cylinder. The pan has a diameter of 35 cm and a height of 0.5 cm.
A chef had enough pizza dough to exactly fill the pan. The dough was in the shape of a sphere.
The pizza was cooked in a hot oven. Once taken out of the oven, the pizza was placed in a dining room.
The temperature, , of the pizza, in degrees Celsius, °C, can be modelled by
where is a constant and is the time, in minutes, since the pizza was taken out of the oven.
When the pizza was taken out of the oven its temperature was 230 °C.
The pizza can be eaten once its temperature drops to 45 °C.
Calculate the volume of this pan.
Find the radius of the sphere in cm, correct to one decimal place.
Find the value of .
Find the temperature that the pizza will be 5 minutes after it is taken out of the oven.
Calculate, to the nearest second, the time since the pizza was taken out of the oven until it can be eaten.
In the context of this model, state what the value of 19 represents.
Abdallah owns a plot of land, near the river Nile, in the form of a quadrilateral ABCD.
The lengths of the sides are and angle .
This information is shown on the diagram.
The formula that the ancient Egyptians used to estimate the area of a quadrilateral ABCD is
.
Abdallah uses this formula to estimate the area of his plot of land.
Show that correct to the nearest metre.
Calculate angle .
Find the area of ABCD.
Calculate Abdallah’s estimate for the area.
Find the percentage error in Abdallah’s estimate.
A water container is made in the shape of a cylinder with internal height cm and internal base radius cm.

The water container has no top. The inner surfaces of the container are to be coated with a water-resistant material.
The volume of the water container is .
The water container is designed so that the area to be coated is minimized.
One can of water-resistant material coats a surface area of .
Write down a formula for , the surface area to be coated.
Express this volume in .
Write down, in terms of and , an equation for the volume of this water container.
Show that .
Find .
Using your answer to part (e), find the value of which minimizes .
Find the value of this minimum area.
Find the least number of cans of water-resistant material that will coat the area in part (g).
A factory packages coconut water in cone-shaped containers with a base radius of 5.2 cm and a height of 13 cm.
The factory designers are currently investigating whether a cone-shaped container can be replaced with a cylinder-shaped container with the same radius and the same total surface area.
Find the slant height of the cone-shaped container.
Find the slant height of the cone-shaped container.
Show that the total surface area of the cone-shaped container is 314 cm2, correct to three significant figures.
Find the height, , of this cylinder-shaped container.
The factory director wants to increase the volume of coconut water sold per container.
State whether or not they should replace the cone-shaped containers with cylinder‑shaped containers. Justify your conclusion.
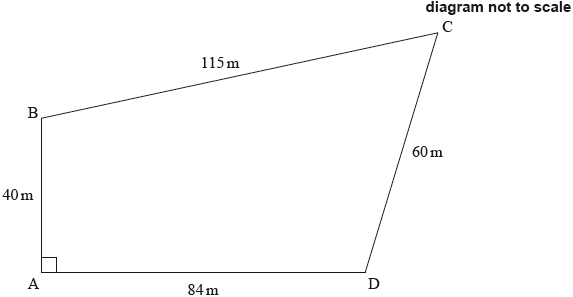
An archaeological site is to be made accessible for viewing by the public. To do this, archaeologists built two straight paths from point A to point B and from point B to point C as shown in the following diagram. The length of path AB is 185 m, the length of path BC is 250 m, and angle is 125°.
The archaeologists plan to build two more straight paths, AD and DC. For the paths to go around the site, angle is to be made equal to 85° and angle is to be made equal to 70° as shown in the following diagram.
Find the size of angle .
Find the size of angle .
The diagram shows the straight line . Points , and are points on .
is the midpoint of .
Line is perpendicular to and passes through point .
The point is on .
Find the gradient of .
Find the coordinates of point .
Find the equation of . Give your answer in the form , where .
Find the value of .
Find the distance between points and .
Given that the length of is , find the area of triangle .
Farmer Brown has built a new barn, on horizontal ground, on his farm. The barn has a cuboid base and a triangular prism roof, as shown in the diagram.
The cuboid has a width of 10 m, a length of 16 m and a height of 5 m.
The roof has two sloping faces and two vertical and identical sides, ADE and GLF.
The face DEFL slopes at an angle of 15° to the horizontal and ED = 7 m .
The roof was built using metal supports. Each support is made from five lengths of metal AE, ED, AD, EM and MN, and the design is shown in the following diagram.
ED = 7 m , AD = 10 m and angle ADE = 15° .
M is the midpoint of AD.
N is the point on ED such that MN is at right angles to ED.
Farmer Brown believes that N is the midpoint of ED.
Calculate the area of triangle EAD.
Calculate the total volume of the barn.
Calculate the length of MN.
Calculate the length of AE.
Show that Farmer Brown is incorrect.
Calculate the total length of metal required for one support.
A manufacturer makes trash cans in the form of a cylinder with a hemispherical top. The trash can has a height of 70 cm. The base radius of both the cylinder and the hemispherical top is 20 cm.
A designer is asked to produce a new trash can.
The new trash can will also be in the form of a cylinder with a hemispherical top.
This trash can will have a height of H cm and a base radius of r cm.
There is a design constraint such that H + 2r = 110 cm.
The designer has to maximize the volume of the trash can.
Write down the height of the cylinder.
Find the total volume of the trash can.
Find the height of the cylinder, h , of the new trash can, in terms of r.
Show that the volume, V cm3 , of the new trash can is given by
.
Using your graphic display calculator, find the value of r which maximizes the value of V.
The designer claims that the new trash can has a capacity that is at least 40% greater than the capacity of the original trash can.
State whether the designer’s claim is correct. Justify your answer.
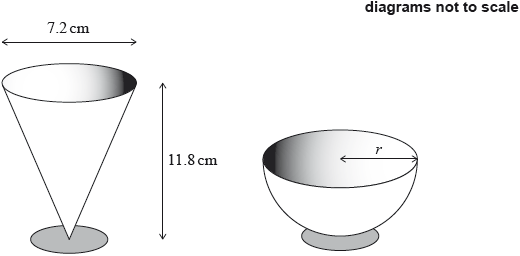
A restaurant serves desserts in glasses in the shape of a cone and in the shape of a hemisphere. The diameter of a cone shaped glass is 7.2 cm and the height of the cone is 11.8 cm as shown.
The volume of a hemisphere shaped glass is .
The restaurant offers two types of dessert.
The regular dessert is a hemisphere shaped glass completely filled with chocolate mousse. The cost, to the restaurant, of the chocolate mousse for one regular dessert is $1.89.
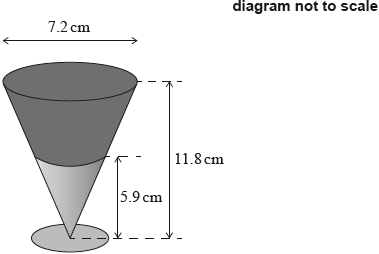
The special dessert is a cone shaped glass filled with two ingredients. It is first filled with orange paste to half of its height and then with chocolate mousse for the remaining volume.
The cost, to the restaurant, of of orange paste is $7.42.
A chef at the restaurant prepares 50 desserts; regular desserts and special desserts. The cost of the ingredients for the 50 desserts is $111.44.
Show that the volume of a cone shaped glass is , correct to 3 significant figures.
Calculate the radius, , of a hemisphere shaped glass.
Find the cost of of chocolate mousse.
Show that there is of orange paste in each special dessert.
Find the total cost of the ingredients of one special dessert.
Find the value of .
Let . The line is tangent to the graph of at .
can be expressed in the form r u.
The direction vector of is .
Find the gradient of .
Find u.
Find the acute angle between and .
Find .
Hence, write down .
Hence or otherwise, find the obtuse angle formed by the tangent line to at and the tangent line to at .
A farmer owns a plot of land in the shape of a quadrilateral ABCD.
and angle .
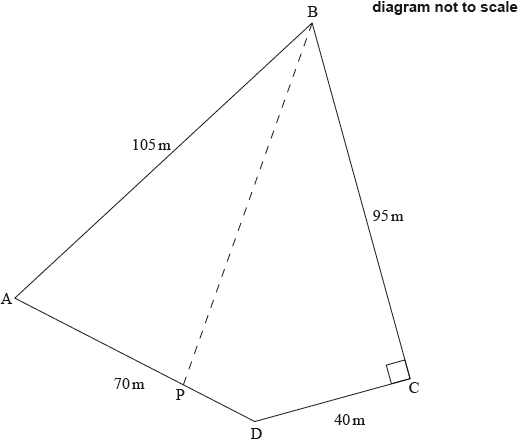
The farmer wants to divide the land into two equal areas. He builds a fence in a straight line from point B to point P on AD, so that the area of PAB is equal to the area of PBCD.
Calculate
the length of BD;
the size of angle DAB;
the area of triangle ABD;
the area of quadrilateral ABCD;
the length of AP;
the length of the fence, BP.
The base of an electric iron can be modelled as a pentagon ABCDE, where:
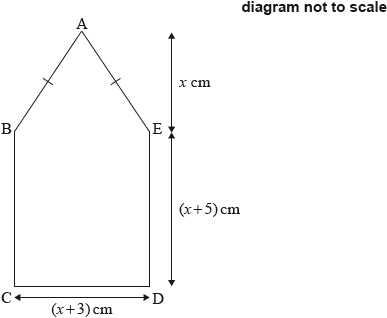
Insulation tape is wrapped around the perimeter of the base of the iron, ABCDE.
F is the point on AB such that . A heating element in the iron runs in a straight line, from C to F.
Write down an equation for the area of ABCDE using the above information.
Show that the equation in part (a)(i) simplifies to .
Find the length of CD.
Show that angle , correct to one decimal place.
Find the length of the perimeter of ABCDE.
Calculate the length of CF.
The Maxwell Ohm Company is designing a portable Bluetooth speaker. The speaker is in the shape of a cylinder with a hemisphere at each end of the cylinder.
The dimensions of the speaker, in centimetres, are illustrated in the following diagram where is the radius of the hemisphere, and is the length of the cylinder, with and .
The Maxwell Ohm Company has decided that the speaker will have a surface area of .
The quality of sound from the speaker will improve as increases.
Write down an expression for , the volume (cm3) of the speaker, in terms of , and .
Write down an equation for the surface area of the speaker in terms of , and .
Given the design constraint that , show that .
Find .
Using your answer to part (d), show that is a maximum when is equal to .
Find the length of the cylinder for which is a maximum.
Calculate the maximum value of .
Use your answer to part (f) to identify the shape of the speaker with the best quality of sound.
Haraya owns two triangular plots of land, and . The length of is , is and is . The size of is and is .
The following diagram shows this information.
Haraya attaches a long rope to a vertical pole at point .
Find the length of .
Find the size of .
Calculate the area of the triangular plot of land .
Determine whether the rope can extend into the triangular plot of land, . Justify your answer.
Consider the points A(−3, 4, 2) and B(8, −1, 5).
A line L has vector equation . The point C (5, , 1) lies on line L.
Find .
Find the value of .
Show that .
Find the angle between and .
Find the area of triangle ABC.
The vector equation of line is given by r .
Point P is the point on that is closest to the origin. Find the coordinates of P.
A communication tower, T, produces a signal that can reach cellular phones within a radius of 32 km. A straight road passes through the area covered by the tower’s signal.
The following diagram shows a line representing the road and a circle representing the area covered by the tower’s signal. Point R is on the circumference of the circle and points S and R are on the road. Point S is 38 km from the tower and RŜT = 43˚.
Let SR = . Use the cosine rule to show that .
Two points P and Q have coordinates (3, 2, 5) and (7, 4, 9) respectively.
Let = 6i − j + 3k.
Find .
Find .
Find the angle between PQ and PR.
Find the area of triangle PQR.
Hence or otherwise find the shortest distance from R to the line through P and Q.
The following diagram shows quadrilateral ABCD.
Find DB.
Find DC.
Let .
Find .
Let . Find .
The following diagram shows a circle, centre O and radius mm. The circle is divided into five equal sectors.
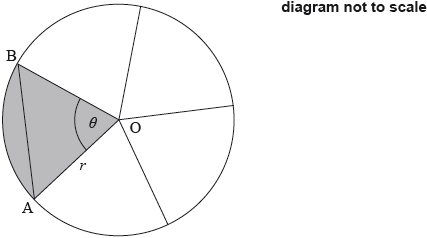
One sector is OAB, and .
The area of sector AOB is .
Write down the exact value of in radians.
Find the value of .
Find AB.
OAB is a sector of the circle with centre O and radius , as shown in the following diagram.
The angle AOB is radians, where .
The point C lies on OA and OA is perpendicular to BC.
Find the area of triangle OBC in terms of and θ.
The following diagram shows a circle with centre O and radius 40 cm.

The points A, B and C are on the circumference of the circle and .
Find the length of arc ABC.
Find the perimeter of sector OABC.
Find the area of sector OABC.
The following diagram shows the chord [AB] in a circle of radius 8 cm, where .
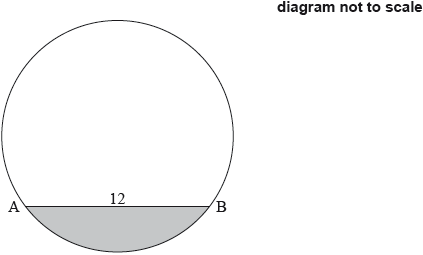
Find the area of the shaded segment.
A ship is sailing north from a point A towards point D. Point C is 175 km north of A. Point D is 60 km north of C. There is an island at E. The bearing of E from A is 055°. The bearing of E from C is 134°. This is shown in the following diagram.
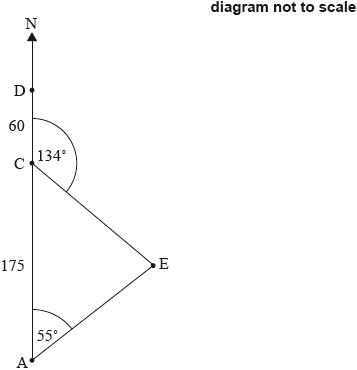
When the ship reaches D, it changes direction and travels directly to the island at 50 km per hour. At the same time as the ship changes direction, a boat starts travelling to the island from a point B. This point B lies on (AC), between A and C, and is the closest point to the island. The ship and the boat arrive at the island at the same time. Find the speed of the boat.