IB Questionbank

User interface language: English | Español

Date	May 2018	Marks available	2	Reference code	18M.2.AHL.TZ2.H_10
Level	Additional Higher Level	Paper	Paper 2	Time zone	Time zone 2
Command term	Sketch	Question number	H_10	Adapted from	N/A

Question

Consider the expression $f\left( x \right) = {\text{tan}}\left( {x + \frac{\pi }{4}} \right){\text{cot}}\left( {\frac{\pi }{4} - x} \right)$ .

The expression $f\left( x \right)$ can be written as $g\left( t \right)$ where $t = {\text{tan}}\,x$ .

Let $\alpha$ , β be the roots of $g\left( t \right) = k$ , where 0 < $k$ < 1.

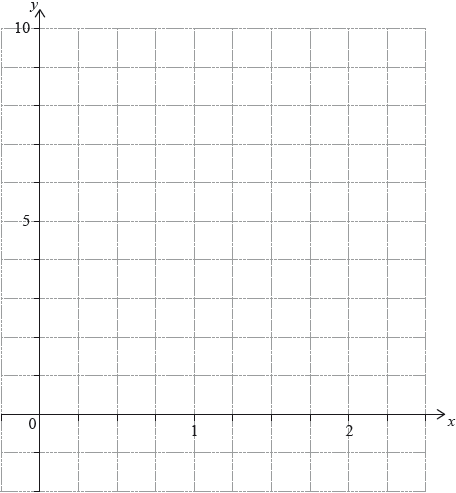
Sketch the graph of $y = f\left( x \right)$ for $- \frac{{5\pi }}{8} \leqslant x \leqslant \frac{\pi }{8}$ .

[2]

a.i.

With reference to your graph, explain why $f$ is a function on the given domain.

[1]

a.ii.

Explain why $f$ has no inverse on the given domain.

[1]

a.iii.

Explain why $f$ is not a function for $- \frac{{3\pi }}{4} \leqslant x \leqslant \frac{\pi }{4}$ .

[1]

a.iv.

Show that $g\left( t \right) = {\left( {\frac{{1 + t}}{{1 - t}}} \right)^2}$ .

[3]

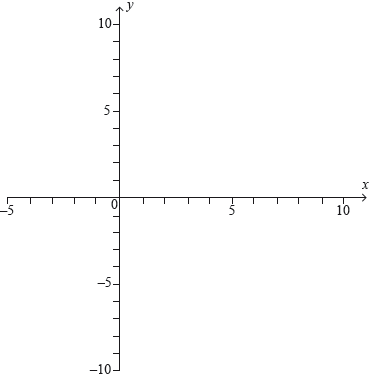
Sketch the graph of $y = g\left( t \right)$ for t ≤ 0. Give the coordinates of any intercepts and the equations of any asymptotes.

[3]

Find $\alpha$ and β in terms of $k$ .

[5]

d.i.

Show that $\alpha$ + β < −2.

[2]

d.ii.

Markscheme

A1A1

A1 for correct concavity, many to one graph, symmetrical about the midpoint of the domain and with two axes intercepts.

Note: Axes intercepts and scales not required.

A1 for correct domain

[2 marks]

a.i.

for each value of $x$ there is a unique value of $f\left( x \right)$ A1

Note: Accept “passes the vertical line test” or equivalent.

[1 mark]

a.ii.

no inverse because the function fails the horizontal line test or equivalent R1

Note: No FT if the graph is in degrees (one-to-one).

[1 mark]

a.iii.

the expression is not valid at either of $x = \frac{\pi }{4}\,\,\left( {{\text{or}} - \frac{{3\pi }}{4}} \right)$ R1

[1 mark]

a.iv.

METHOD 1

$f\left( x \right) = \frac{{{\text{tan}}\left( {x + \frac{\pi }{4}} \right)}}{{{\text{tan}}\left( {\frac{\pi }{4} - x} \right)}}$ M1

$= \frac{{\frac{{{\text{tan}}\,x + {\text{tan}}\,\frac{\pi }{4}}}{{1 - {\text{tan}}\,x\,{\text{tan}}\,\frac{\pi }{4}}}}}{{\frac{{{\text{tan}}\,\frac{\pi }{4} - {\text{tan}}\,x}}{{1 + {\text{tan}}\,\frac{\pi }{4}{\text{tan}}\,x}}}}$ M1A1

$= {\left( {\frac{{1 + t}}{{1 - t}}} \right)^2}$ AG

METHOD 2

$f\left( x \right) = {\text{tan}}\left( {x + \frac{\pi }{4}} \right){\text{tan}}\left( {\frac{\pi }{2} - \frac{\pi }{4} + x} \right)$ (M1)

$= {\text{ta}}{{\text{n}}^2}\left( {x + \frac{\pi }{4}} \right)$ A1

$g\left( t \right) = {\left( {\frac{{{\text{tan}}\,x + {\text{tan}}\,\frac{\pi }{4}}}{{1 - {\text{tan}}\,x\,{\text{tan}}\,\frac{\pi }{4}}}} \right)^2}$ A1

$= {\left( {\frac{{1 + t}}{{1 - t}}} \right)^2}$ AG

[3 marks]

for t ≤ 0, correct concavity with two axes intercepts and with asymptote $y$ = 1 A1

t intercept at (−1, 0) A1

$y$ intercept at (0, 1) A1

[3 marks]

METHOD 1

$\alpha$ , β satisfy $\frac{{{{\left( {1 + t} \right)}^2}}}{{{{\left( {1 - t} \right)}^2}}} = k$ M1

$1 + {t^2} + 2t = k\left( {1 + {t^2} - 2t} \right)$ A1

$\left( {k - 1} \right){t^2} - 2\left( {k + 1} \right)t + \left( {k - 1} \right) = 0$ A1

attempt at using quadratic formula M1

$\alpha$ , β $= \frac{{k + 1 \pm 2\sqrt k }}{{k - 1}}$ or equivalent A1

METHOD 2

$\alpha$ , β satisfy $\frac{{1 + t}}{{1 - t}} = \left( \pm \right)\sqrt k$ M1

$t + \sqrt k t = \sqrt k - 1$ M1

$t = \frac{{\sqrt k - 1}}{{\sqrt k + 1}}$ (or equivalent) A1

$t - \sqrt k t = - \left( {\sqrt k + 1} \right)$ M1

$t = \frac{{\sqrt k + 1}}{{\sqrt k - 1}}$ (or equivalent) A1

so for eg, $\alpha = \frac{{\sqrt k - 1}}{{\sqrt k + 1}}$ , β $= \frac{{\sqrt k + 1}}{{\sqrt k - 1}}$

[5 marks]

d.i.

$\alpha$ + β $= 2\frac{{\left( {k + 1} \right)}}{{\left( {k - 1} \right)}}\,\left( { = - 2\frac{{\left( {1 + k} \right)}}{{\left( {1 - k} \right)}}} \right)$ A1