5.4 Further Integration

1a 1b 2a 2b 2c 3a 3b 4a 4b 5a 5b 5c 6 7a 7b 7c 8a 8b 8c 9 10a 10b 11

Question 1a

Marks: 3

Consider the function $f$ defined by $f (x) = (x^{2} - 3 x + 2) (x + 2), - 3 \leq x \leq \frac{5}{4} .$

Find the indefinite integral

\int (x^{2} - 3 x + 2) (x + 2) d x

Key Concepts

Integrating Powers of x

Question 1b

Marks: 4

Use your answer to part (a) to calculate the area of the region enclosed by the graph of

y = f (x)

and the

x

-axis.

Key Concepts

Definite Integrals

Question 2a

Marks: 2

Find the indefinite integral for

\int \sin (\frac{\sqrt{3}}{2} x) d x

Key Concepts

Integrating Trig Functions

Question 2b

Marks: 2

Find the indefinite integral for

\int \frac{7}{e^{4 x - 9}} d x

Key Concepts

Integrating e^x & 1/x

Question 2c

Marks: 2

Find an expression for

y

given that

\frac{d y}{d x} = \cos (2 (\frac{π}{8} - x))

Key Concepts

Integrating Trig Functions

Question 3a

Marks: 2

Find the indefinite integral

\int - \frac{7}{5 x} d x

Key Concepts

Integrating e^x & 1/x
Integrating Composite Functions (ax+b)

Question 3b

Marks: 3

Find an expression for given that

\frac{d y}{d x} = x e^{x^{2} - 2}

and also that

y = 3

when

x = - \sqrt{2}

Key Concepts

Integrating Composite Functions (ax+b)
Finding the Constant of Integration

Question 4a

Marks: 3

Find the indefinite integral for

\int (\sqrt{3 x} + \frac{5}{\sqrt[3]{8 x}}) d x

Key Concepts

Integrating Powers of x
Laws of Indices

Question 4b

Marks: 3

Find the indefinite integral for

\int \frac{{(8 x)}^{\frac{2}{3}} - 5 x^{\frac{1}{6}}}{x \sqrt[3]{x}} d x

Key Concepts

Integrating Powers of x
Laws of Indices

Question 5a

Marks: 4

Find the indefinite integral

\int \frac{x + 2 x^{2}}{(1 - x^{2}) (2 + x^{2})} d x

Key Concepts

Reverse Chain Rule

Question 5b

Marks: 5

Let

g' (x) = \frac{\cos (\ln x)}{x}

for

x > 0 .

Find

g (x)

given that

g (1) = π

Key Concepts

Reverse Chain Rule
Finding the Constant of Integration

Question 5c

Marks: 4

Show that

\int \tan x d x = \ln |\frac{1}{\cos x}| + c

Key Concepts

Trigonometry - Simple Identities
Reverse Chain Rule

Question 6

Marks: 6

A curve with equation $y = f (x)$ is such that

$\frac{d y}{d x} = \frac{k}{(1 + \sin π x) (1 - \sin π x)}$

where $k$ is a real constant.

Given that the curve passes through the points $(0, - 3)$ and $(- \frac{1}{4}, - π)$ , find $f (x)$ .

Key Concepts

Integrating Trig Functions
Finding the Constant of Integration

Question 7a

Marks: 2

Explain why

\frac{1}{\tan θ} = \frac{\cos θ}{\sin θ}

Key Concepts

Trigonometry - Simple Identities

Question 7b

Marks: 7

Use definite integration, along with the result from part (a), to show that

\int_{0}^{\sqrt{\frac{π}{6}}} \frac{x}{\tan (x^{2} - \frac{2 π}{3})} d x = - \frac{1}{2} \ln (\frac{\sqrt{3}}{2})

Key Concepts

Reverse Chain Rule
Definite Integrals

Question 7c

Marks: 1

Using your knowledge of the natural logarithm function, explain (without using your GDC) why the value of the integral found in part (b) is a positive number.

Key Concepts

Logarithmic Functions & Graphs

Question 8a

Marks: 4

The diagram below shows the graph of the function $f$ which is defined by

$f (x) = x \sin 2 x, 0 \leq x \leq \frac{3 π}{2}$

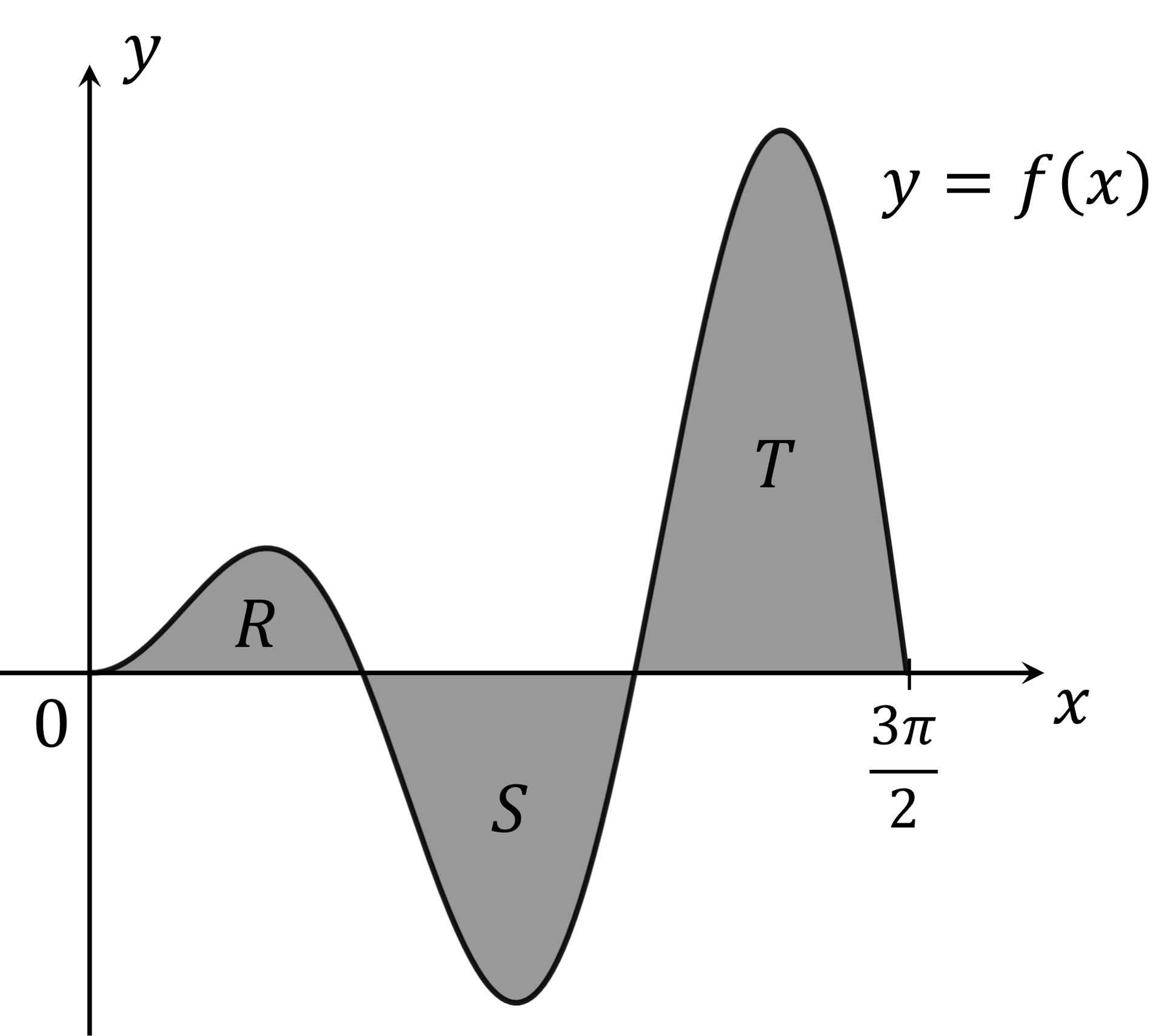
mi-q8a-5-4-ib-ai-hl-further-integration-very-hard_dig

The shaded region in the diagram is the region enclosed by the $x$ -axis and the graph of $y = f (x)$ . The three sub-parts of the shaded region are denoted by R, S and T, as shown.

(a) Find the value of

$\int_{0}^{\frac{3 π}{2}} x \sin 2 x d x$

Key Concepts

Definite Integrals using GDC

Question 8b

Marks: 5

Find the individual areas of each of the three sub-parts R, S and T of the shaded region.

Key Concepts

Area Under a Curve Basics
Negative Integrals

Question 8c

Marks: 4

Compare the sum of the answers in part (b) to the answer in part (a) and comment on the result.

Key Concepts

Negative Integrals

Question 9

Marks: 8

The diagram below depicts the design for a new company logo. The upper border of the logo is formed by a part of the curve with equation $y = 9 - x^{2}$ , while the lower border of the logo is formed by a part of the curve with equation $y = \frac{1}{8} x^{2} - 5$ . As shown in the diagram, the logo is divided into a shaded part and an unshaded part by a part of the curve with equation $y = \frac{(9 - x^{2}) (2 x - 1)}{10}$ .

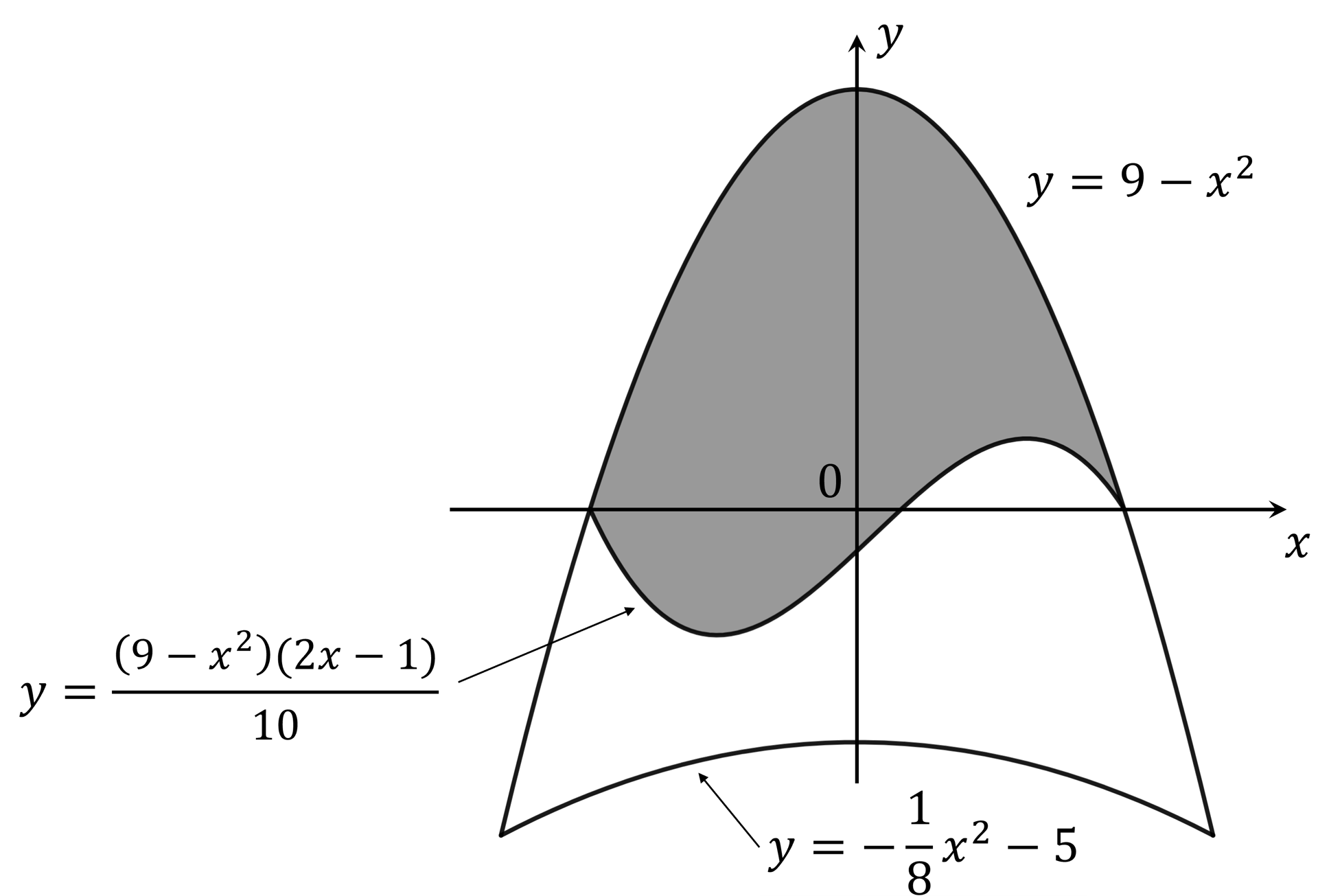
mi-q9-5-4-ib-ai-hl-further-integration-very-hard_dig

Find the percentage of the total area of the logo that is shaded.

Key Concepts

Area Under a Curve Basics
Negative Integrals

Question 10a

Marks: 9

The following diagram shows a part of the graph of the curve with equation $y^{2} = k (1600 - 25 {(x - 8)}^{2})$ , where $k > 0$ is a constant. The point marked A is the vertex of the curve. Region $R$ is the region enclosed by the curve and the $x$ -axis, for the part of the curve where $y$ is non-negative. Region $S$ is the region enclosed by the curve, the positive $y$ -axis, and the line through point A with gradient zero.

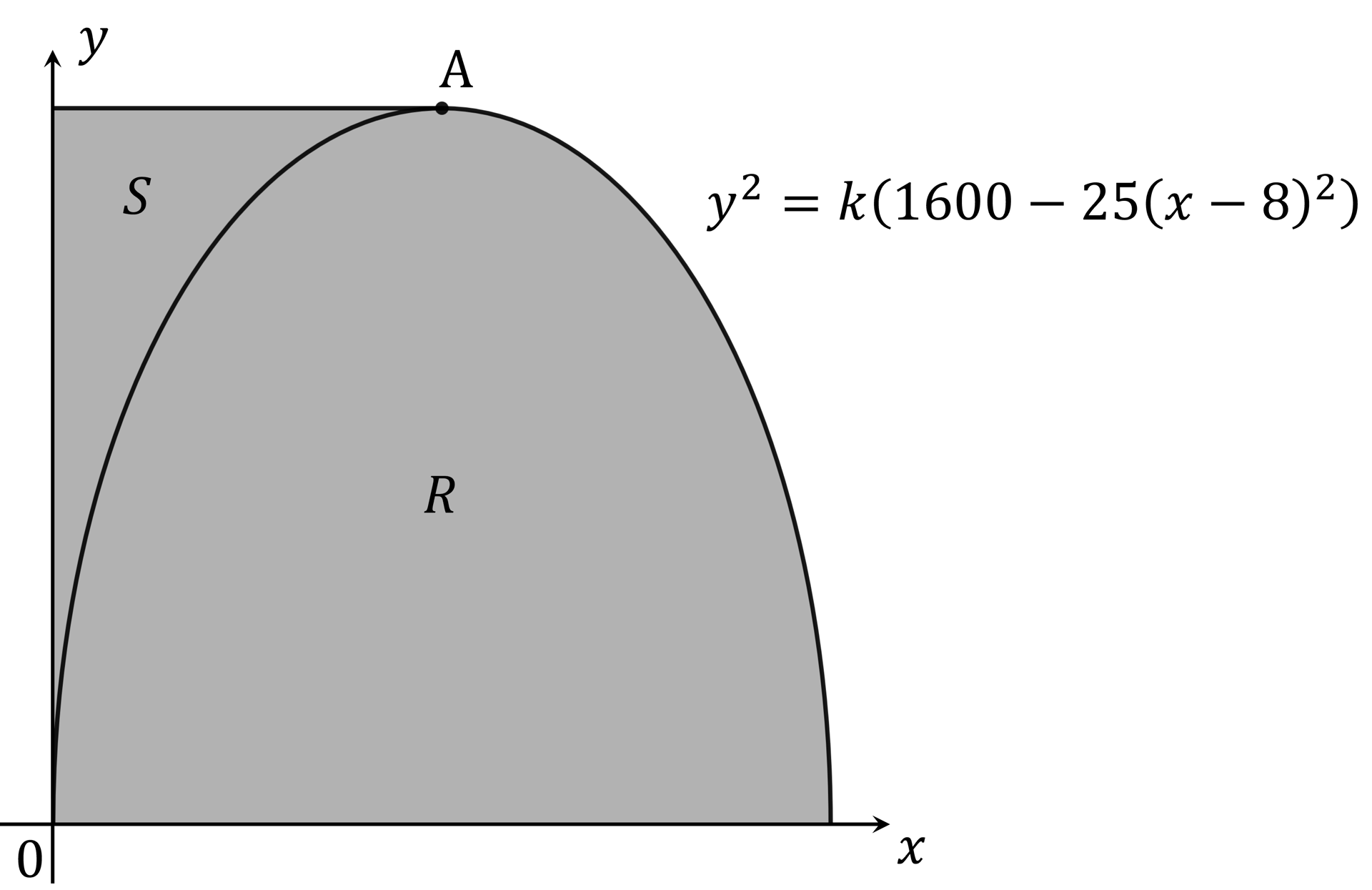
mi-q10a-5-4-ib-ai-hl-further-integration-very-hard-2_dig

When region $R$ is rotated $2 π$ radians about the $x$ -axis, the resultant solid of revolution has a volume equal to $\frac{12800 π}{3} {units}^{3}$ .

Find the area of region

S

by calculating an area between the curve and the

y

-axis.

Key Concepts

Volumes of Revolution Around x-axis
Area Between Curve & y-axis

Question 10b

Marks: 4

Find the area of region S by calculating an area between the curve and the

x

-axis. Confirm that this matches your answer to part (a).

Key Concepts

Area Under a Curve Basics

Question 11

Marks: 8

The diagram below shows the cross-section of a goldfish bowl to be produced by Pieseize Manufacturing, a specialist company supplying products for goldfish enthusiasts.

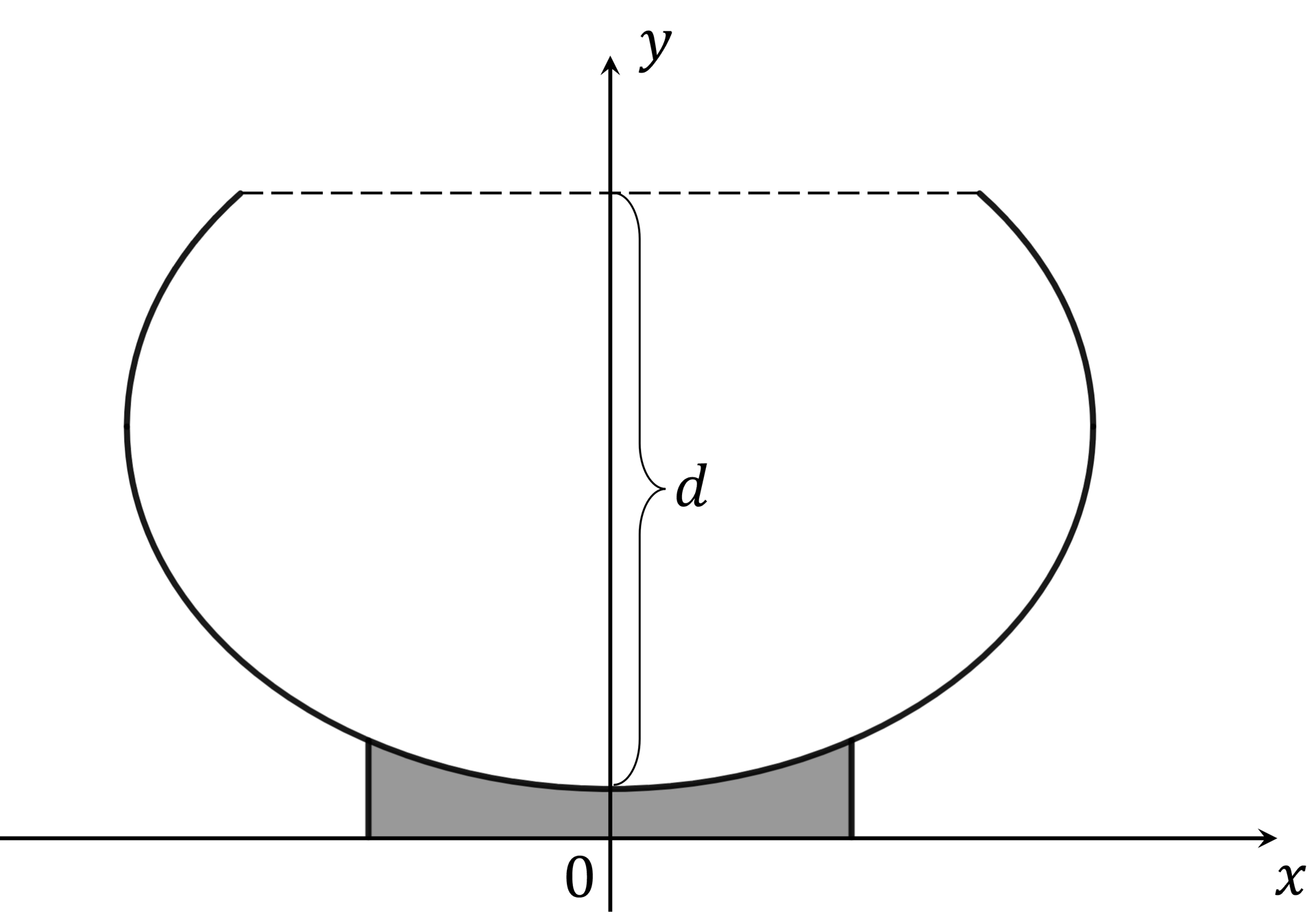
q12-5-9-ib-aa-hl-advanced-integration-very-hard-maths_diagram

The glass part of the bowl sits on a solid base, indicated by the shaded region on the diagram. The cross-section of the glass part of the bowl is symmetrical about the $y$ -axis, and may be described by the curve with equation

$\frac{x^{2}}{400} + \frac{{(y - 17)}^{2}}{225} = 1$

The dashed horizontal line represents the diameter of the open top of the fishbowl. The maximum depth of the fishbowl, measured along the $y$ -axis from the diameter of the open top to where the glass part of the bowl meets the base, is indicated by $d$ in the diagram. All coordinates are expressed in centimetres, and for purposes of answering this question the thickness of the glass sides of the bowl may be regarded as negligible.

The owner of the company, Skodyn Pieseize, is extremely superstitious and is obsessed with the number 23. Therefore he insists that the capacity of the glass part of this new fishbowl must be exactly 23 litres. Find the value of $d$ that satisfies this requirement.

Key Concepts

Volumes of Revolution Around y-axis
Modelling with Volumes of Revolution

DP IB Maths: AI HL

Topic Questions

5.4 Further Integration

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Key Concepts

Resources

Members

Company

Quick Links